Curated OER
Functions - Intro and Inverses
Define the terms domain, range, function, vertical limit test, and linear function notation with your class. They can then, graph several equations applying the vertical line test to determine which are and which are not functions, write...
University of Northern Texas
Continuity
Continue a study of calculus by using a slideshow to introduce the concept of continuity. After defining the term, the presentation provides examples of functions that are discontinuous and introduces different types...
Curated OER
Is There a Limit to Which Side You Can Take?
Calculus learners find the limit of piecewise functions at a value. They find the limit of piecewise functions as x approaches a given value. They find the limit of linear, quadratic, exponential, and trigonometric piecewise functions.
Curated OER
Continuity and Limits
In this continuity and limits worksheet, students solve and complete 4 different types of problems. First, they determine which of the limits shown exist and then, evaluate them. They also find which functions are continuous at a given...
Royal Society of Chemistry
Functional Groups
Looking for a highly functional tool to teach young chemists functional groups? Engage the class with a series of logic-based games. Users identify 12 different functional groups by name and formula to work their way through challenging...
National Security Agency
Multiple Representations of Limits
After an introductory activity to demonstrate the theory of a limit, additional activities approach a limit from graphical, numerical, and algebraic methods. The activity looks at the multiple ways of understanding and evaluating a...
Curated OER
Finding the Limit: Piecewise Functions and Graphs
This pre-calculus worksheet is short, yet challenging. High schoolers calculate the limit of piecewise functions, rational functions, and graphs as x approaches a number from the positive or negative side. There are four questions.
Curated OER
Explorations 5: Introduction to Limits
In this limit worksheet, students find the interval values of given functions. They identify constants, and plot graphs. This three-page worksheet contains approximately 20 problems.
Curated OER
Worksheet 10 - Limits
In this limits worksheets, students graph tangent lines and determine the slope of a secant line. This one-page worksheet contains six multi-step problems.
Curated OER
Worksheet 4: Graphs and One-Sided Limits
In this math worksheet, learners answer 6 questions regarding functions, graphs, one-sided limits, intercepts, vehicle speed and wheel rotation.
Curated OER
Quiz: Logarithmic Functions
Explore logarithmic functions, and have pupils solve 10 different problems that include various logarithmic functions. First, they find the domain of a given function and then the range. They also consider a graph that has a limited...
Curated OER
Worksheet 26 - Functions & Logarithms
In this function and logarithms worksheet, students find the domain and range of functions, use the properties of logs to solve equations. This one-page worksheet contains nine multi-step problems.
Curated OER
Worksheet 5: Property Limits and the Squeeze Theorem
In this math worksheet, students answer 6 questions regarding given limits in a table of data, properties of limits and the Squeeze Theorem.
Curated OER
Worksheet 2: Graphs, Functions and Derivatives
In this math worksheet, young scholars answer 7 questions having to do with graphing derivatives of functions, rectilinear motion, speed and distance.
Curated OER
Math 155 - Fall 2002 Worksheet 3: Continuity
In this continuity worksheet, students solve 5 short answer problems about continuity. Students determine where functions are continuous and find the limits of functions at points of discontinuity.
CK-12 Foundation
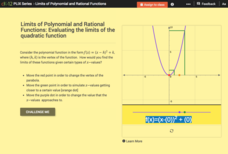
Limits of Polynomial and Rational Functions: Evaluating the Limits of the Quadratic Function
Push an engaging resource to the limit. The interactive allows learners to find a limit on quadratic functions graphically. Using sliders, pupils set the x-value for the limit and to move values from the left and right toward the limit.
Curated OER
Open Sets, Limits, Continuity
In this open sets worksheet, students give characteristics of an open set under given conditions. They graph functions and define limits. This two-page worksheet contains 3 multi-step problems with explanations.
Radford University
Skate Ramp
Going up and down makes a more exciting ride. Pupils recall what they know about continuity and limits of functions. Working in groups, classmates design a skateboard ramp that meets a given set of criteria, using at least three...
Shodor Education Foundation
InteGreat
Hands-on investigation of Riemann sums becomes possible without intensive arithmetic gymnastics with this interactive lesson plan. Learners manipulate online graphing tools to develop and test theories about right, left, and...
Flipped Math
Calculus AB/BC - Connecting Multiple Representations of Limits
It's time to put it all together. Viewers of an informative video learn to connect multiple representations of limits. Given algebraic, tabular, and graphical representations of a linear piecewise function, they determine whether a limit...
Mathematics Vision Project
Module 7: Modeling with Functions
The sky's the limit of what you create when combining functions! The module begins with a review of transformations of parent functions and then moves to combining different function types using addition, subtraction, and...
Alabama Learning Exchange
Building Functions: Composition of Functions
Hammer away at building different types of functions. An engaging lesson builds on learners' knowledge of domain and range to create an understanding of composite functions. Young scholars learn to write composite functions...
Concord Consortium
Rational and Not So Rational Functions
Do not cross the line while graphing. Provided with several coordinate axes along with asymptotes, pupils determine two functions that will fit the given restrictions. Scholars then determine other geometrical relationships of asymptotes...
West Contra Costa Unified School District
Graphing Exponential Functions
Once you know how to graph y = b^x, the sky's the limit. Young mathematicians learn to graph basic exponential functions and identify key features, and then graph functions of the form f(x) = ab^(x – h) + k from the function f(x) = b^x.