Radford University
Parallelogram Task
These floors were made for learning. Pupils use coordinate planes drawn on the classroom floor using tape to investigate parallelograms. They measure lengths, angles, and slopes to identify properties of parallelograms.
Radford University
Finding a Location for Your Kitchen Island
Where is the best place to work? Small groups map out a kitchen on a coordinate plane and find the centers of the work triangle to find the best place to install an island. The teams use algebraic methods to locate the center by finding...
Corbett Maths
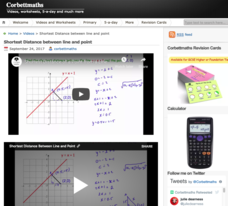
Shortest Distance between Line and Point
A short video shows how to find the shortest distance between a line and a point on the coordinate plane. Using the fact the shortest distance is the perpendicular, the presenter shows the class how to find the intersection of the two...
Corbett Maths
Coordinates and Shapes
Where is the point? Given three points on the coordinate plane, the video walks through the steps to find the location of the fourth point to create a given special quadrilateral. The narrator first determines the coordinates of the...
Mathematics Vision Project
Module 6: Connecting Algebra and Geometry
A geometry module connects algebraic reasoning to geometry. It challenges scholars to investigate the slope criteria for parallel and perpendicular lines, prove theorems involving coordinate geometry, and write equations for circles and...
101 Questions
Best Midpoint
Develop a strong understanding of what it means to be a midpoint. Learners analyze the angles, coordinates, and lengths of segments and their approximated midpoints. They use their analyses to develop a formula to rank four attempts at...
101 Questions
Best Square
If you're a square, be the best square you can be! Young scholars develop a formula to determine the four points that make the best square that considers the area, perimeter, and other dimensions. They use their formulas to rank attempts...
101 Questions
Best Circle
Drawing the perfect circle is harder than one would think! What makes a circle a circle and how can you define that with a formula? Young mathematicians devise their own methods of analyzing the imperfections of circle drawings. Using...
101 Questions
Best Triangle
What makes an equilateral triangle equilateral? It turns out it's much more than just the side lengths! Learners analyze four different triangles to determine the best equilateral triangle. They create a formula that they later use to...
CK-12 Foundation
Polygon Classification in the Coordinate Plane
Classify this resource into the "Use" pile. Scholars use an interactive coordinate plane to plot polygons given coordinates for the vertices. They use properties to classify each polygon and answer a few challenge questions regarding the...
CK-12 Foundation
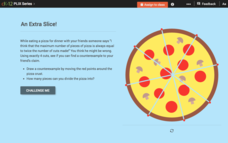
Conjectures and Counterexamples: An Extra Slice!
Class members will eat up an enticing interactive that lets users change the location of cuts made into a pizza to adjust the number of created slices. They create a counterexample for a conjecture on the number of slices.
Mathematics Vision Project
Connecting Algebra and Geometry
Connect algebra and geometry on the coordinate plane. The eighth unit in a nine-part integrated course has pupils develop the distance formula from the Pythagorean Theorem. Scholars prove geometric theorems using coordinates...
Virginia Department of Education
Circles in the Coordinate Plane
Make the connection between the distance formula and the equation of a circle. The teacher presents a instructional activity on how to use the distance formula to derive the equation of the circle. Pupils transform circles on the...
Virginia Department of Education
Distance and Midpoint Formulas
Small groups work through two guided activities to derive the distance and midpoint formulas for the coordinate plane. The activities begin with concrete examples and move to abstract.
Illustrative Mathematics
Coordinates of Equilateral Triangles
Can it be constructed? The task poses the question whether it is possible to have an equilateral triangle with its vertices located at integer coordinates. Pupils work with their knowledge of trigonometric ratios and the Pythagorean...
Mathematics Assessment Project
Classifying Equations of Parallel and Perpendicular Lines
Parallel parking might be difficult, but finding parallel lines is fairly simple. In this lesson plan, learners first complete an assessment task involving parallel and perpendicular lines in the coordinate plane. Individuals then take...
Mathematics Assessment Project
Square
Don't be a square! Young mathematicians determine the slope and length of a line segment. They then prove whether four given coordinate points form a square.
Curated OER
Expressing Geometric Properties with Equations
Algebra and geometry are not interchangeable. Demonstrate why not with a series of problems that deal with the equations of circles and equations of lines that meet specific criteria.
EngageNY
Geometry Module 5: End-of-Module Assessment
The lessons are complete. Learners take an end-of-module assessment in the last installment of a 23-part module. Questions contain multiple parts, each assessing different aspects of the module.
EngageNY
Equations for Tangent Lines to Circles
Don't go off on a tangent while writing equations of tangent lines! Scholars determine the equations for tangent lines to circles. They attempt both concrete and abstract examples, such as a tangent line to the unit circle through...
EngageNY
The Distance from a Point to a Line
What is the fastest way to get from point A to line l? A straight perpendicular line! Learners use what they have learned in the previous lessons in this series and develop a formula for finding the shortest distance from...
EngageNY
Motion Along a Line – Search Robots Again
We can mathematically model the path of a robot. Learners use parametric equations to find the location of a robot at a given time. They compare the paths of multiple robots looking for parallel and perpendicular relationships and...
EngageNY
Analytic Proofs of Theorems Previously Proved by Synthetic Means
Prove theorems through an analysis. Learners find the midpoint of each side of a triangle, draw the medians, and find the centroid. They then examine the location of the centroid on each median discovering there is a 1:2 relationship....
EngageNY
Dividing Segments Proportionately
Fractions, ratios, and proportions: what do they have to do with segments? Scholars discover the midpoint formula through coordinate geometry. Next, they expand on the formula to apply it to dividing the segment into different...